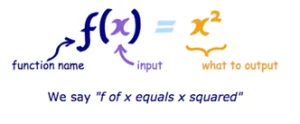Updated: Feb 7
All the topics that are on the exam are listed below. There are also drop-down menus for each of the broad topics in which more specific content is listed. IF you feel comfortable with these topics, you should be fully prepared for the exam!
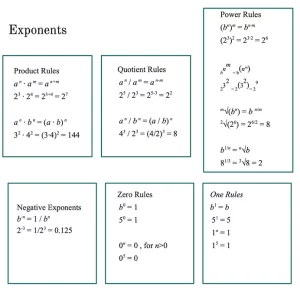
PEMDAS (Parenthesis, Exponents, Division, Addition, Subtraction)
– Like terms: Terms whose variables (such as x or y) with any exponents (such as the 2 in x2) are the same. Examples: 7x and 2x are like terms because they are both “x”.
– REMINDER: Always check what they are asking for before selecting your answer. For example, the questions may ask “what is x + 4” as opposed to just “what is x”.
– 3 Quadratic Identities (unfactored to factored form) (x2-y2)=(x+y)(x-y) x2+2xy+y2=(x+y)2 x2-2xy+y2=(x-y)2
Plugin hypothetical numbers for each variable!
Choose numbers that are easy to work with (ex: 100, 10, 4, etc.)
METHOD 1: Substitution
2x + 4y = 6 AND y = 5 —–> 2x + 4(5) = 6
MEDTHOD 2: Elimination
2x + 4y = 6 Subtract these two equations to eliminate the “x”:
2x – y = – 9
5 y = 15
y = 3
METHOD 3: Set Equal
y = 2x + 3 AND y = 4x + 1 ——> 2x + 3 = 4x + 1
Break the equation into two equations (positive and negative)
Absolute Value Equations will always have two answers!
| 2x + 4 | = 6
2x + 4 = + 6 2x + 4 = – 6
2x = 2 2x = – 10
x = 1 x = -5
y = 2x + 3 AND y = 4x + 1 ——> 2x + 3 = 4x + 1
– Pretend the inequality sign is an equal sign and solve for the variable!
– When you divide or multiply by a negative, FLIP THE SIGN!
-6x – 9 > 3
Add 9
-6x > 12
Divide by -6 AND flip the sign
x < -2
– The “x” is Just a Place-Holder! It is just there to show us where the input goes and what happens to it. It could be anything!
– The value of a function is undefined when the denominator is equal to zero (#36 C Test 1)
– Sequences are all about finding the pattern!
ax^2 + bx + c = 0
METHOD 1: Factoring
– Find two numbers that multiply to the last term and add to the middle term
METHOD 2: Quadratic Formula

The Discriminant
– The discriminant is everything under the radical in the quadratic equation = b^2 – 4ac
– If the discriminant is POSITIVE, then there are 2 real roots (“roots” is another word for “solutions” when equations are written in ax^2+by+c = 0 form).
– If the discriminant is ZERO, then there is 1 real root.
– If the discriminant is NEGATIVE, then there are no real roots. (#13 C Test 6)
– Know how to complete the square
– These are similar to functions! Think about the symbol as a function.

– Binomial addition involving constants and i by combining like terms (adding and subtracting complex numbers)
– Multiplying by the conjugate of the denominator with complex numbers (#11 Test 2)
– Polynomial Remainder Theorem (#29 C Test 1) (#7 NC Test 3)
– Divisible by 2 = last digit 0, 2, 4, 6, 8?
– Divisible by 3 = sum of digits divisible by 3?
– Divisible by 4 = last 2 digits divisible by 4?
– Divisible by 5 = last digit 0 or 5?
– Divisible by 6 = follow rule 2 and 3?
– Divisible by 8 = last three digits divisible by 8?
– Divisible by 9 = sum of the digits divisible by 9?
– Divisible by 10 = last digit 0?
– Percentage = (Part/Whole)
– Percent Change = (Difference/Original) x 100)
– Special Triangles (3/4/5, 5/12/13, 30/60/90, 45/45/90)
– Pythagorean theorem is used to find the third side of a right triangle. It is ONLY for right triangles. NOTE: Hypotenuse is always the longest side.
– The largest side is opposite the largest angle. The smallest side is opposite the smallest angle.
– All three angles add up to 180 degrees!
– The sides of similar triangles all have the same respective proportions. (#17 NC Test 1, #18 NC Test 2)
– The Third Side Rule for Triangles (a-b) < c < (a+b) if c represents the “third side” and b and a represent the lengths of the other two sides.
– The proportion of distance that you travel along the hypotenuse of a right triangle is equal to the proportion of distance that you travel along both legs. (#16 NC Test 4)
– The Circle Proportionality Formula (Slice/Area = Arc/Circumference = Measure of Inner Angle/360)
– The arc measure formed by an angle with its vertex on a circle is double the measure of the angle. (#36 C Test 5)
– The equation of a circle with center (h,k) and radius r: (x-h)2 + (y-k)2= r2 (#24 C Test 1)
Surface Area of a Cube = 6s2
Area of a triangle = 1/2 ab sin C
Most of the formulas for 2D and 3D Objects are given on the first page of the math section of the SAT. Remember to review that cheat sheet before the exam to know what formulas are given. Unfortunately, the ACT does not provide any formulas.
– SOH CAH TOA
– Sin x = Cos (90-x) (#19 NC Test 1)
– The vertex of a parabola is located at the midpoint of its x-intercepts (#12 NC Test 3)
– The vertex (h,k) form of a parabola: a(x-h)^2 + k
– When an upward projectile reaches its highest point, its velocity is zero.
– When an upward projectile lands, its height is zero. – To find the intersections of two lines, set them equal to one another (#13 test 4)
– In a system of linear equations, there is no solution if the slopes of the two lines are the same (parallel) and the y-intercept is different. (see #9 Test 3) Conversely, there are infinitely many solutions is the slopes of the two lines are the same and the y-intercept is also the same (#20 NC Test 2)
– The “zeroes” or “roots” of a function are the x-coordinates where it crosses the x-axis (and where the y value outputs zero).
– A polynomial of Nth degree has at most N-1 changes in direction.
– Domain and Range
– Parallel Lines and Transversals (#36 Test 1)
– The Formula for a Line (standard y=mx+b format as well as point-slope format: y-y1 = m(x-x1), and the slope equation (y2-y1) / (x2-x1) ).
– Positive and Negative Associations in Graphs (#5 C Test #1)
A line extends forever in both directions.
A line segment has two endpoints.
A ray is a part of a line that has one endpoint and goes on infinitely in only one direction.
A midpoint is the middle of the line. To solve for the midpoint, take the average of the x value and the average of the y value.
A line measures 180degrees.
Types of angles to know:
– opposite angles
– supplementary angles
– opposite interior angles
– complimetary angles
– π radians = 180 degrees (#19 NC Test 2)
Probability = (Desired Possibilities / Total Possibilities)
Permutation = arranging the objects or numbers in order
Combinations = selecting objects or numbers from a group, in which order doesn’t matter.
Simple interest is based on the principal amount of a loan or deposit. In contrast,
compound interest is based on the principal amount and the interest that accumulates on it in every period.
Distance = Rate x Time (#38 C Test 5, #9 C Test 3)
If you are in search of a tutor for the SAT & ACT, don’t hesitate to reach out to Prep For Success. Our team is happy to help prepare you for your exam!
You can reach out at PrepForSuccessTutors@gmail.com or (732) 485.1539 or (201) 566.5943.